背景
AVL 树是一棵平衡的二叉查找树,于 1962 年,G. M.Adelson-Velsky 和 E. M. Landis 在他们的论文《An algorithm for the organization of information》中发表。
所谓的平衡之意,就是树中任意一个结点下左右两个子树的高度差不超过 1。(本文对于树的高度约定为:空结点高度是 0,叶子结点高度是 1。)

那 AVL 树和普通的二叉查找树有何区别呢?如图,如果我们插入的是一组有序上升或下降的数据,则一棵普通的二叉查找树必然会退化成一个单链表,其查找效率就降为 O(n)。而 AVL 树因其平衡的限制,可以始终保持 O(logn) 的时间复杂度。
具体实现与代码分析
在我们进行完插入或删除操作后,很可能会导致某个结点失去平衡,那么我们就需要把失衡结点旋转一下,使其重新恢复平衡。
经过分析,不管是插入还是删除,它们都会有四种失衡的情况:左左失衡,右右失衡,左右失衡,右左失衡。因此每次遇到失衡时,我们只需判断一下是哪个失衡,再对其进行相对应的恢复平衡操作即可。
好,下面以插入操作为例,来看下这四种失衡的庐山真面目。(以下统一约定:红色结点为新插入结点,y 结点为失衡结点)
(1)左左失衡

所谓的左左,即 "失衡结点" 的左子树比右子树高 2,左孩子下的左子树比右子树高 1。
我们只需对 "以 y 为根的子树" 进行 "左左旋转 (ll_rotate)" 即可。一次旋转后,恢复平衡。
Node * AVL::ll_rotate(Node * y)
{
Node * x = y->left;
y->left = x->right;
x->right = y;
y->height = max(get_height(y->left), get_height(y->right)) + 1;
x->height = max(get_height(x->left), get_height(x->right)) + 1;
return x;
}
(2)右右失衡

所谓的右右,即 "失衡结点" 的右子树比左子树高 2,右孩子下的右子树比左子树高 1。
我们只需对 "以 y 为根的子树" 进行 "右右旋转 (rr_rotate)" 即可。一次旋转后,恢复平衡。
Node * AVL::rr_rotate(Node * y)
{
Node * x = y->right;
y->right = x->left;
x->left = y;
y->height = max(get_height(y->left), get_height(y->right)) + 1;
x->height = max(get_height(x->left), get_height(x->right)) + 1;
return x;
}
(3)左右失衡

所谓的左右,即 "失衡结点" 的左子树比右子树高 2,左孩子下的右子树比左子树高 1。
观察发现,若先对 "以 x 为根的子树" 进行 "右右旋转 (rr_rotate)",此时 "以 y 为根的子树" 恰好符合 "左左失衡",所以再进行一次 "左左旋转 (ll_rotate)"。两次旋转后,恢复平衡。
Node * AVL::lr_rotate(Node * y)
{
Node * x = y->left;
y->left = rr_rotate(x);
return ll_rotate(y);
}
(4)右左失衡

所谓的右左,即 "失衡结点" 的右子树比左子树高 2,右孩子下的左子树比右子树高 1。
观察发现,若先对 "以 x 为根的子树" 进行 "左左旋转 (ll_rotate)",此时 "以 y 为根的子树" 恰好符合 "右右失衡",所以再进行一次 "右右旋转 (rr_rotate)"。两次旋转后,恢复平衡。
Node * AVL::rl_rotate(Node * y)
{
Node * x = y->right;
y->right = ll_rotate(x);
return rr_rotate(y);
}
插入操作
插入成功后,在递归回溯时依次对经过的结点判断是否失衡,若失衡就需要对其进行对应的旋转操作使其恢复平衡,在这期间,原先作为一棵子树的根结点就会因为旋转被替换,因此设置insert_real( )返回的是新根结点,这样就可以实时更新根结点。
插入操作实现代码如下:
int AVL::get_height(Node * node)
{
if (node == nullptr)
return 0;
return node->height;
}
int AVL::get_balance(Node * node)
{
if (node == nullptr)
return 0;
return get_height(node->left) - get_height(node->right);
}
Node * AVL::insert_real(int key, Node * node)
{
if (node == nullptr)
return new Node(key);
if (key < node->key)
node->left = insert_real(key, node->left);
else if (key > node->key)
node->right = insert_real(key, node->right);
else
return node;
node->height = max(get_height(node->left), get_height(node->right)) + 1;
int balance = get_balance(node);
// 左左失衡
if (balance > 1 && get_balance(node->left) > 0)
return ll_rotate(node);
// 右右失衡
if (balance < -1 && get_balance(node->right) < 0)
return rr_rotate(node);
// 左右失衡
if (balance > 1 && get_balance(node->left) < 0)
return lr_rotate(node);
// 右左失衡
if (balance < -1 && get_balance(node->right) > 0)
return rl_rotate(node);
return node;
}
void AVL::insert(int key)
{
header->left = insert_real(key, header->left);
}
查找操作
Node * AVL::find_real(int key, Node * node)
{
if (node == nullptr)
return nullptr;
if (key < node->key)
return find_real(key, node->left);
else if (key > node->key)
return find_real(key, node->right);
else
return node;
}
Node * AVL::find(int key)
{
return find_real(key, header->left);
}
删除操作
删除操作的四种失衡情况和插入操作一样,读者可以参考前文。下面是删除操作的实现代码:
Node * AVL::erase_real(int key, Node * node)
{
if (node == nullptr)
return node;
if (key < node->key)
node->left = erase_real(key, node->left);
else if (key > node->key)
node->right = erase_real(key, node->right);
else
{
if (node->left && node->right)
{
// 找到后继结点
Node * x = node->right;
while (x->left)
x = x->left;
// 后继直接复制
node->key = x->key;
// 转化为删除后继
node->right = erase_real(x->key, node->right);
}
else
{
Node * t = node;
node = node->left ? node->left : node->right;
deletet;
if (node == nullptr)
return nullptr;
}
}
node->height = max(get_height(node->left), get_height(node->right)) + 1;
int balance = get_balance(node);
// 左左失衡
if (balance > 1 && get_balance(node->left) >= 0) // 需要加等号
return ll_rotate(node);
// 右右失衡
if (balance < -1 && get_balance(node->right) <= 0) // 需要加等号
return rr_rotate(node);
// 左右失衡
if (balance > 1 && get_balance(node->left) < 0)
return lr_rotate(node);
// 右左失衡
if (balance < -1 && get_balance(node->right) > 0)
return rl_rotate(node);
return node;
}
void AVL::erase(int key)
{
header->left = erase_real(key, header->left);
}
完整代码
/**
*
* author : 刘毅(Limer)
* date : 2017-08-17
* mode :C++
*/
#include
#include
using namespace std;
struct Node
{
int key;
int height;
Node * left;
Node * right;
Node(int key = 0)
{
this->key = key;
this->height = 1;
this->left = this->right = nullptr;
}
};
class AVL
{
private:
Node * header;
private:
Node * ll_rotate(Node * y);
Node * rr_rotate(Node * y);
Node * lr_rotate(Node * y);
Node * rl_rotate(Node * y);
void destroy(Node * node);
int get_height(Node * node);
int get_balance(Node * node);
Node * insert_real(int key, Node * node);
Node * find_real(int key, Node * node);
Node * erase_real(int key, Node * node);
void in_order(Node * node);
public:
AVL();
~AVL();
void insert(int key);
Node * find(int key);
void erase(int key);
void print();
};
Node * AVL::ll_rotate(Node * y)
{
Node * x = y->left;
y->left = x->right;
x->right = y;
y->height = max(get_height(y->left), get_height(y->right)) + 1;
x->height = max(get_height(x->left), get_height(x->right)) + 1;
return x;
}
Node * AVL::rr_rotate(Node * y)
{
Node * x = y->right;
y->right = x->left;
x->left = y;
y->height = max(get_height(y->left), get_height(y->right)) + 1;
x->height = max(get_height(x->left), get_height(x->right)) + 1;
return x;
}
Node * AVL::lr_rotate(Node * y)
{
Node * x = y->left;
y->left = rr_rotate(x);
return ll_rotate(y);
}
Node * AVL::rl_rotate(Node * y)
{
Node * x = y->right;
y->right = ll_rotate(x);
return rr_rotate(y);
}
void AVL::destroy(Node * node)
{
if (node == nullptr)
return;
destroy(node->left);
destroy(node->right);
delete node;
}
int AVL::get_height(Node * node)
{
if (node == nullptr)
return 0;
return node->height;
}
int AVL::get_balance(Node * node)
{
if (node == nullptr)
return 0;
return get_height(node->left) - get_height(node->right);
}
Node * AVL::insert_real(int key, Node * node)
{
if (node == nullptr)
return new Node(key);
if (key < node->key)
node->left = insert_real(key, node->left);
else if (key > node->key)
node->right = insert_real(key, node->right);
else
return node;
node->height = max(get_height(node->left), get_height(node->right)) + 1;
int balance = get_balance(node);
// 左左失衡
if (balance > 1 && get_balance(node->left) > 0)
return ll_rotate(node);
// 右右失衡
if (balance < -1 && get_balance(node->right) < 0)
return rr_rotate(node);
// 左右失衡
if (balance > 1 && get_balance(node->left) < 0)
return lr_rotate(node);
// 右左失衡
if (balance < -1 && get_balance(node->right) > 0)
return rl_rotate(node);
return node;
}
Node * AVL::find_real(int key, Node * node)
{
if (node == nullptr)
return nullptr;
if (key < node->key)
return find_real(key, node->left);
else if (key > node->key)
return find_real(key, node->right);
else
return node;
}
Node * AVL::erase_real(int key, Node * node)
{
if (node == nullptr)
return node;
if (key < node->key)
node->left = erase_real(key, node->left);
else if (key > node->key)
node->right = erase_real(key, node->right);
else
{
if (node->left && node->right)
{
// 找到后继结点
Node * x = node->right;
while (x->left)
x = x->left;
// 后继直接复制
node->key = x->key;
// 转化为删除后继
node->right = erase_real(x->key, node->right);
}
else
{
Node * t = node;
node = node->left ? node->left : node->right;
delete t;
if (node == nullptr)
return nullptr;
}
}
node->height = max(get_height(node->left), get_height(node->right)) + 1;
int balance = get_balance(node);
// 左左失衡
if (balance > 1 && get_balance(node->left) >= 0) // 需要加等号
return ll_rotate(node);
// 右右失衡
if (balance < -1 && get_balance(node->right) <= 0) // 需要加等号
return rr_rotate(node);
// 左右失衡
if (balance > 1 && get_balance(node->left) < 0)
return lr_rotate(node);
// 右左失衡
if (balance < -1 && get_balance(node->right) > 0)
return rl_rotate(node);
return node;
}
void AVL::in_order(Node * node)
{
if (node == nullptr)
return;
in_order(node->left);
cout << node->key << " ";
in_order(node->right);
}
AVL::AVL()
{
header = new Node(0);
}
AVL::~AVL()
{
destroy(header->left);
delete header;
header = nullptr;
}
void AVL::insert(int key)
{
header->left = insert_real(key, header->left);
}
Node * AVL::find(int key)
{
return find_real(key, header->left);
}
void AVL::erase(int key)
{
header->left = erase_real(key, header->left);
}
void AVL::print()
{
in_order(header->left);
cout << endl;
}
int main()
{
AVL avl;
// test "insert"
avl.insert(7);
avl.insert(2);
avl.insert(1); avl.insert(1);
avl.insert(5);
avl.insert(3);
avl.insert(6);
avl.insert(4);
avl.insert(9);
avl.insert(8);
avl.insert(11); avl.insert(11);
avl.insert(10);
avl.insert(12);
avl.print(); // 1 2 3 4 5 6 7 8 9 10 11 12
// test "find"
Node * p = nullptr;
cout << ((p = avl.find(2)) ? p->key : -1) << endl; // 2
cout << ((p = avl.find(100)) ? p->key : -1) << endl; // -1
// test "erase"
avl.erase(1);
avl.print(); // 2 3 4 5 6 7 8 9 10 11 12
avl.erase(9);
avl.print(); // 2 3 4 5 6 7 8 10 11 12
avl.erase(11);
avl.print(); // 2 3 4 5 6 7 8 10 12
return 0;
}
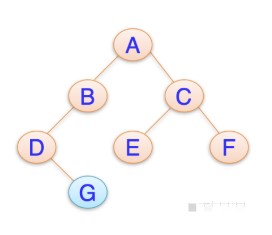
起初构造的 AVL 树为下图:

总结
和二叉查找树相比,AVL 树的特点是时间复杂度更稳定,但缺点也是很明显的。
插入操作中,至多需要一次恢复平衡操作,递归回溯的量级为 O(logn)。有一点需要我们注意,在对第一个失衡结点进行恢复平衡后,递归回溯就应该立即停止(因为失衡结点的父亲及其祖先们肯定都是处于平衡状态的)。
但让 "递归的回溯" 中途停止,不好实现,所以我上面的编码程序都不可避免的会继续回溯,直到整棵树的根结点,而这些回溯都是没有必要的。(谢谢 LLL 的提醒,若在结点中增设父亲结点,就可以解决递归回溯的问题)
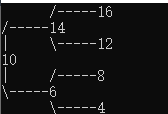
删除操作中,若存在失衡,则至少需要一次恢复平衡操作,递归回溯的量级亦为 O(logn)。与插入操作不同,当对第一个失衡结点恢复平衡后,它的父亲或者是它的祖先们也可能是非平衡的(见下图,删除 1),所以删除操作的回溯很有必要。

没有参照物对比的探讨是没有意义的,所以此文就止于此吧,有兴趣的朋友可以看下我后面《红黑树》及《AVL 树与红黑树的对比》的文章。
- AVL
+关注
关注
0文章
14浏览量
10021 - 二叉树
+关注
关注
0文章
74浏览量
12265
原文标题:一文读懂 AVL 树
文章出处:【微信号:TheAlgorithm,微信公众号:算法与数据结构】欢迎添加关注!文章转载请注明出处。
发布评论请先登录
相关推荐
二叉树层次遍历算法的验证
红黑树(Red Black Tree)是一种自平衡的二叉搜索树
二叉树操作的相关知识和代码详解
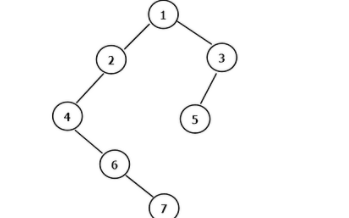




 AVL 树和普通的二叉查找树的详细区别分析
AVL 树和普通的二叉查找树的详细区别分析










评论